本章主要讲解正弦定理、余弦定理、海伦公式、Stewart's Theorem。
本文在没有特殊说明时,默认在 \(\triangle ABC\) 中:
- \(a\) 为角 \(A\) 对边,\(b\) 为角 \(B\) 对边,\(c\) 为角 \(C\) 对边。
- \(r\) 为内切圆半径(inradius),\(R\) 为外接圆半径(circumradius)。
- \([ABC]\) 为 \(\triangle ABC\) 的面积。
- \(s\) 为半周长(semiperimeter)。
余弦定理(law of cosines)
书上给了一个运用圆幂定理(the Power of a Point Theorem)的方法,但是太过复杂,事实上直接解三角形即可证。
\[c^2 = a^2 + b^2 - 2ab \cos C \]\[\cos C = \dfrac {a^2 + b^2 - c^2} {2ab} \]一般用于:
- 已知 SAS 求 S
- 已知 SSS 求 A
经典结论
- \(c^2 < a^2 + b^2\) 当且仅当 \(\angle C\) 为锐角(acute angle)
- \(c^2 > a^2 + b^2\) 当且仅当 \(\angle C\) 为钝角(obtuse angle)
正弦定理(law of sines)
\[\dfrac a {\sin A} = \dfrac b {\sin B} = \dfrac c {\sin C} = 2R \]一般用于:
- 已知 ASA / AAS 求 S
- 已知 SAS / SSA 求 A
经典结论
小角对小边,大角对大边。(\(\angle_A > \angle_B\) 当且仅当 \(a > b\))
正切定理(law of tangents)
\[\dfrac {a-b} {a+b} = \dfrac {\tan \frac {A-B} 2} {\tan \frac {A+B} 2} \]三角形的面积公式
\[[ABC] = \dfrac 1 2 ab \sin C = \dfrac {abc} {4R} = rs = \dfrac {a^2 \sin B \sin C} {2 \sin A} = 2R^2 \sin A \sin B \sin C \]海伦公式(Heron's formula)
\[[ABC] = \sqrt {s(s-a)(s-b)(s-c)} \]展开形式:
\[[ABC] = \dfrac 1 4 \sqrt {(a+b+c)(-a+b+c)(a-b+c)(a+b-c)} \]Stewart's Theorem
(A \(man\) and his \(dad\) put a \(bomb\) in the \(sink\))
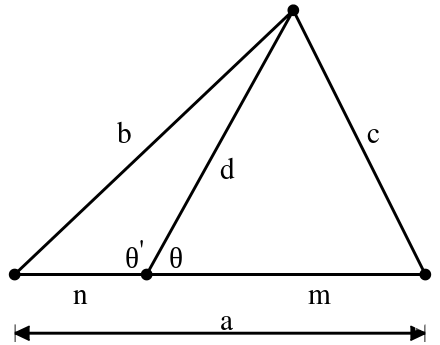
中线长公式
当 \(m=n\) 时:
\[d = \sqrt {\dfrac 1 2 b^2 + \dfrac 1 2 c^2 - \dfrac 1 4 a^2} \]角平分线长公式
当 \(\dfrac b n = \dfrac c m\) 时:
\[d = \sqrt {bc \left(1 - \dfrac {a^2} {(b+c)^2}\right)} \]另一个公式:(习题 No. 53)
\[d = 2 \cos \frac C 2 \dfrac 1 {\frac 1 a + \frac 1 b} \]课后习题
No. 46
Problem
Show that in \(\triangle ABC\),
\[\sin \dfrac A 2 = \sqrt {\dfrac {(s-b)(s-c)} {bc}} \]Solution
\[\begin{aligned} RHS &= \sqrt {\dfrac {\frac {a-b+c} 2 \frac {a+b-c} 2} {bc}} \\ &= \dfrac 1 2 \sqrt {\dfrac {a^2 -b^2 - c^2 + 2bc} {bc}} \\ &= \dfrac 1 2 \sqrt{2 - 2 \cos A} \\ &= \dfrac 1 2 \sqrt {2 - 2 \left(\cos^2 \frac A 2 - \sin^2 \frac A 2\right)} \\ &= \dfrac 1 2 \sqrt {4 \sin^2 \frac A 2} \\ &= \sin \frac A 2 \end{aligned}\]No. 57
Problem
Prove that if \(\alpha, \beta, \gamma > 0\) and \(\alpha + \beta + \gamma = \pi\), then \(\sin 2\alpha + \sin 2\beta + \sin 2\gamma = 4 \sin\alpha \sin\beta \sin\gamma\).
Solution
令 \(\alpha, \beta, \gamma\) 为一个三角形的三个角,记为 \(A,B,C\)。
\[\begin{aligned} RHS &= 4 \sin A \sin B \sin C \\ &= 4 \dfrac {abc} {8R^3} \\ &= \dfrac 2 {R^2} [ABC] \end{aligned}\]\[\begin{aligned} LHS &= \sin 2A + \sin 2B + \sin 2C \\ &= 2 (\sin A \cos A + \sin B \cos B + \sin C \cos C) \\ &= \dfrac 2 {R^2} [ABC] \end{aligned}\]LHS 的最后一步可以通过圆周角定理之后作高得出。
标签:Chapter,cos,ABC,frac,dfrac,AoPS,sqrt,sin,Triangles From: https://www.cnblogs.com/AugustLight/p/-/AoPS-Vol2-Chapter-03