[C++]LeetCode1971. 寻找图中是否存在路径
题目描述
Difficulty: 简单
Related Topics: 深度优先搜索, 广度优先搜索, 并查集, 图
有一个具有 n 个顶点的 双向 图,其中每个顶点标记从 0 到 n - 1(包含 0 和 n - 1)。图中的边用一个二维整数数组 edges 表示,其中 edges[i] = [ui, vi] 表示顶点 ui 和顶点 vi 之间的双向边。 每个顶点对由 最多一条 边连接,并且没有顶点存在与自身相连的边。
请你确定是否存在从顶点 source 开始,到顶点 destination 结束的 有效路径 。
给你数组 edges 和整数 n、source 和 destination,如果从 source 到 destination 存在 有效路径 ,则返回 true,否则返回 false 。
示例 1:
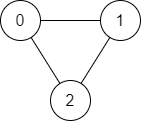
输入:n = 3, edges = [[0,1],[1,2],[2,0]], source = 0, destination = 2
输出:true
解释:存在由顶点 0 到顶点 2 的路径:
- 0 → 1 → 2
- 0 → 2
示例 2:
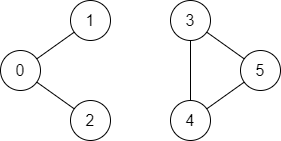
输入:n = 6, edges = [[0,1],[0,2],[3,5],[5,4],[4,3]], source = 0, destination = 5
输出:false
解释:不存在由顶点 0 到顶点 5 的路径.
提示:
- 1 <= n <= 2 * 105
- 0 <= edges.length <= 2 * 105
edges[i].length == 2- 0 <= ui, vi <= n - 1
- ui != vi
0 <= source, destination <= n - 1- 不存在重复边
- 不存在指向顶点自身的边
思路
并查集
用并查集维护连通性,对每条边[u, v],将u、v并入同一集合,最后判断起点与终点是否在相同集合即可。
这里并查集的实现比较简单,时间复杂度为\(O(m+n\log n)\),如果按秩合并/启发式合并复杂度是\(O(m+n\alpha(n))\)。
空间复杂度\(O(n)\)。
Code
Language: C++
class Solution {
static const int N = 2e5 + 5;
int fa[N];
int getfa(int u) {
return fa[u] == u ? u : fa[u] = getfa(fa[u]);
}
public:
bool validPath(int n, vector<vector<int>>& edges, int source, int destination) {
for (int i = 0; i < n; ++i) fa[i] = i;
for (const auto& e : edges) {
if (getfa(e[0]) != getfa(e[1])) fa[getfa(e[0])] = getfa(e[1]);
}
return getfa(source) == getfa(destination);
}
};