首页 > 其他分享 >【数据结构】之链表详解

【数据结构】之链表详解

时间：2024-10-17 18:52:54浏览次数：3  
标签：Node current head next 链表 详解 数据结构 节点

链表是一种常用的数据结构，它是一种线性数据结构，但与数组不同，它并非连续存储数据，而是通过指针将数据节点连接起来。每个节点都包含数据域和指向下一个节点的指针域。这种结构赋予链表独特的优势和局限性，使其在某些场景下优于数组，在另一些场景下则相对逊色。本文将深入探讨链表，包括单向链表、双向链表和循环链表，并分析其优缺点。

1. 概念概述

链表由多个节点组成，每个节点包含两个部分：

  • 数据域 (data)：存储实际数据，可以是任何数据类型。

  • 指针域 (next)：指向链表中下一个节点的地址。

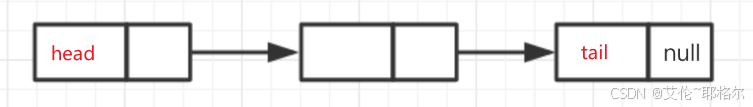
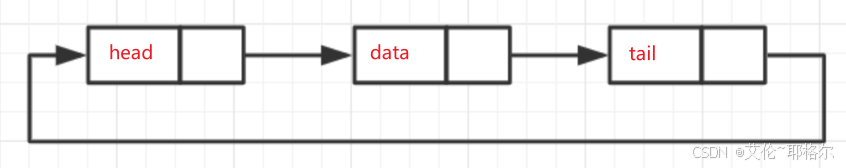
如上图，链表的第一个节点称为头节点 (head)，最后一个节点称为尾节点 (tail)。尾节点的指针域通常指向 null，表示链表的结束。

2. 链表的特点

优点：

  • 动态分配内存： 链表可以动态分配内存，不必事先指定大小，可以根据需要添加或删除节点。

  • 灵活插入和删除： 在链表中间插入或删除节点，只需修改指针即可，时间复杂度为 O(1)。

  • 无需连续内存： 链表的节点可以在内存中分散存储，不需要连续的内存空间。

缺点：

  • 随机访问困难： 链表无法像数组一样通过下标直接访问元素，需要遍历才能找到指定位置的节点。

  • 空间开销： 每个节点都需要额外的指针域存储地址，导致空间开销相对较大。

  • 维护复杂： 链表的操作需要仔细维护指针，容易出错。

3. 链表的 Java 实现

为了更好地理解链表，我们先定义一个简单的节点类：

public class Node {
    // 数据域
    public int data;
    // 指针域
    public Node next;

    // 构造函数
    public Node(int data) {
        this.data = data;
        this.next = null; // 初始化时，指向null
    }
}

接下来，我们将实现一个包含常见方法的单向链表类：

public class SinglyLinkedList {

    // 头节点
    private Node head;

    // 初始化空链表
    public SinglyLinkedList() {
        head = null;
    }

    // 检查链表是否为空
    public boolean isEmpty() {
        return head == null;
    }

    // 获取链表长度
    public int size() {
        int count = 0;
        Node current = head;
        while (current != null) {
            count++;
            current = current.next;
        }
        return count;
    }

    // 在链表头部插入节点
    public void insertAtHead(int data) {
        Node newNode = new Node(data);
        newNode.next = head; // 新节点的next指向原头节点
        head = newNode; // 更新头节点
    }

    // 在链表尾部插入节点
    public void insertAtTail(int data) {
        Node newNode = new Node(data);
        if (isEmpty()) {
            head = newNode; // 空链表，直接将新节点设为头节点
            return;
        }

        Node current = head;
        while (current.next != null) {
            current = current.next; // 遍历到最后一个节点
        }
        current.next = newNode; // 将最后一个节点的next指向新节点
    }

    // 在指定位置插入节点
    public void insertAtIndex(int index, int data) {
        if (index < 0 || index > size()) {
            return; // 非法索引
        }

        if (index == 0) {
            insertAtHead(data); // 如果索引为0，直接调用插入头部方法
            return;
        }

        Node newNode = new Node(data);
        Node current = head;
        for (int i = 0; i < index - 1; i++) {
            current = current.next; // 遍历到指定位置的前一个节点
        }
        newNode.next = current.next; // 新节点的next指向原指定位置的节点
        current.next = newNode; // 指定位置的前一个节点的next指向新节点
    }

    // 删除链表头部的节点
    public void deleteAtHead() {
        if (isEmpty()) {
            return; // 空链表，无需删除
        }
        head = head.next; // 将头节点指向下一个节点
    }

    // 删除链表尾部的节点
    public void deleteAtTail() {
        if (isEmpty()) {
            return; // 空链表，无需删除
        }

        if (head.next == null) { // 只有一个节点的情况
            head = null;
            return;
        }

        Node current = head;
        while (current.next.next != null) {
            current = current.next; // 遍历到倒数第二个节点
        }
        current.next = null; // 将倒数第二个节点的next指向null
    }

    // 删除指定位置的节点
    public void deleteAtIndex(int index) {
        if (index < 0 || index >= size()) {
            return; // 非法索引
        }

        if (index == 0) {
            deleteAtHead(); // 如果索引为0，直接调用删除头部方法
            return;
        }

        Node current = head;
        for (int i = 0; i < index - 1; i++) {
            current = current.next; // 遍历到指定位置的前一个节点
        }
        current.next = current.next.next; // 将指定位置的前一个节点的next指向指定位置的下一个节点
    }

    // 查找链表中第一个值为data的节点
    public Node search(int data) {
        Node current = head;
        while (current != null) {
            if (current.data == data) {
                return current; // 找到节点，返回
            }
            current = current.next; // 继续遍历
        }
        return null; // 未找到，返回null
    }

    // 打印链表
    public void printList() {
        Node current = head;
        while (current != null) {
            System.out.print(current.data + " "); // 打印节点数据
            current = current.next; // 继续遍历
        }
        System.out.println(); // 换行
    }
}

4. 链表的基本操作图解

以上代码示例展示了单向链表的一些基本操作，光看代码看不出所以然，这里通过图示进行详解，包括：

4.1 插入： 在头部、尾部或指定位置插入节点。

#头插法：将新节点插入至第一个节点的位置

a.演示将新节点6插入第一节点位置，也就是插入节点1的位置

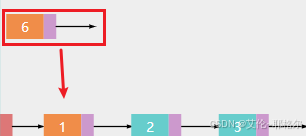
b.先让6的next指向原来的第一个节点，也就是指向节点1

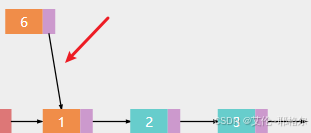
c.然后让head的next指向新插入的节点6，此时原来节点1就会与head自动断开

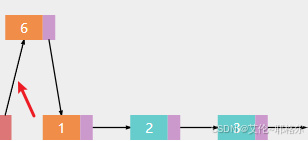
d.成功插入

#尾插法：这里不做直接演示，具体原理是 -> 直接让原本的最后一个节点的next指向要插入的节点，然后再让这个新节点的next指向null，成为新的尾节点。

#中间插入：将新节点插入到链表的中间任意位置

a.将新节点6插入索引1的位置，也就是插入到节点2的位置

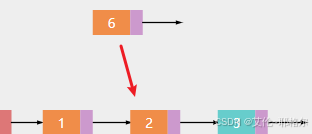
b.先让新节点6的next指向节点2

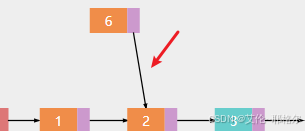
c.然后让节点1指向新节点6，这里有个细节是，插入时，还要知道插入位置的前一个节点的位置

d.成功插入

4.2 删除： 删除头部、尾部或指定位置的节点。

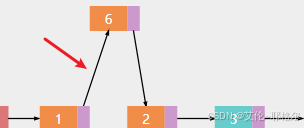
a.删除节点6

 

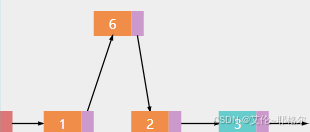
b.让被删除的节点6的前一个节点1，让节点1指向节点2，这里有一个细节是，删除时，你要知道你的前一个节点的位置和后一个节点的位置，在这就是，删除节点6，要知道节点1、2的位置。若是删除最后一个节点，只需让它的前一个节点的next指向null就好

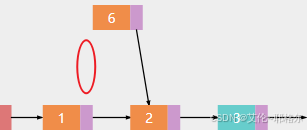
c.删除成功

4.3 查找： 查找链表中值为data的节点。

略

4.4 遍历： 打印所有节点的数据。

略

5. 各个操作的时间复杂度

操作时间复杂度说明
插入头部O(1)只需修改头指针和新节点的指针
插入尾部O(n)需要遍历链表找到尾节点
插入指定位置O(n)需要遍历链表找到指定位置
删除头部O(1)只需修改头指针
删除尾部O(n)需要遍历链表找到尾节点
删除指定位置O(n)需要遍历链表找到指定位置
查找O(n)需要遍历链表查找目标节点
遍历O(n)需要访问所有节点

6. 链表的局限性

尽管链表具有动态性、灵活性和无需连续内存的优势，但它也有一些局限性：

  • 随机访问困难： 无法像数组一样通过下标直接访问元素，需要遍历才能找到指定位置的节点。

  • 空间开销： 每个节点都需要额外的指针域存储地址，导致空间开销相对较大。

  • 维护复杂： 链表的操作需要仔细维护指针，容易出错。

7. 双向链表

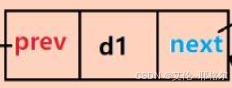
单向链表只能从头节点开始遍历，无法从尾节点反向遍历。双向链表则通过添加一个指向前一个节点的指针域 (prev) 来解决这个问题。

节点组成：

public class DoublyLinkedList {
    // 头节点
    private Node head;
    // 尾节点
    private Node tail;

    public DoublyLinkedList() {
        head = null;
        tail = null;
    }

    // 检查链表是否为空
    public boolean isEmpty() {
        return head == null;
    }

    // 获取链表长度
    public int size() {
        int count = 0;
        Node current = head;
        while (current != null) {
            count++;
            current = current.next;
        }
        return count;
    }

    // 在链表头部插入节点
    public void insertAtHead(int data) {
        Node newNode = new Node(data);
        if (isEmpty()) {
            head = newNode;
            tail = newNode;
        } else {
            newNode.next = head; // 新节点的next指向原头节点
            head.prev = newNode; // 原头节点的prev指向新节点
            head = newNode; // 更新头节点
        }
    }

    // 在链表尾部插入节点
    public void insertAtTail(int data) {
        Node newNode = new Node(data);
        if (isEmpty()) {
            head = newNode;
            tail = newNode;
        } else {
            tail.next = newNode; // 原尾节点的next指向新节点
            newNode.prev = tail; // 新节点的prev指向原尾节点
            tail = newNode; // 更新尾节点
        }
    }

    // 在指定位置插入节点
    public void insertAtIndex(int index, int data) {
        if (index < 0 || index > size()) {
            return; // 非法索引
        }

        if (index == 0) {
            insertAtHead(data); // 如果索引为0，直接调用插入头部方法
            return;
        }

        if (index == size()) {
            insertAtTail(data); // 如果索引等于链表长度，直接调用插入尾部方法
            return;
        }

        Node newNode = new Node(data);
        Node current = head;
        for (int i = 0; i < index - 1; i++) {
            current = current.next; // 遍历到指定位置的前一个节点
        }
        newNode.next = current.next; // 新节点的next指向原指定位置的节点
        newNode.prev = current; // 新节点的prev指向指定位置的前一个节点
        current.next.prev = newNode; // 原指定位置节点的prev指向新节点
        current.next = newNode; // 指定位置的前一个节点的next指向新节点
    }

    // 删除链表头部的节点
    public void deleteAtHead() {
        if (isEmpty()) {
            return; // 空链表，无需删除
        }

        if (head == tail) { // 只有一个节点的情况
            head = null;
            tail = null;
            return;
        }

        head = head.next; // 将头节点指向下一个节点
        head.prev = null; // 更新头节点的prev指向null
    }

    // 删除链表尾部的节点
    public void deleteAtTail() {
        if (isEmpty()) {
            return; // 空链表，无需删除
        }

        if (head == tail) { // 只有一个节点的情况
            head = null;
            tail = null;
            return;
        }

        tail = tail.prev; // 将尾节点指向前一个节点
        tail.next = null; // 更新尾节点的next指向null
    }

    // 删除指定位置的节点
    public void deleteAtIndex(int index) {
        if (index < 0 || index >= size()) {
            return; // 非法索引
        }

        if (index == 0) {
            deleteAtHead(); // 如果索引为0，直接调用删除头部方法
            return;
        }

        if (index == size() - 1) {
            deleteAtTail(); // 如果索引为最后一个节点，直接调用删除尾部方法
            return;
        }

        Node current = head;
        for (int i = 0; i < index; i++) {
            current = current.next; // 遍历到指定位置的节点
        }
        current.prev.next = current.next; // 指定位置前一个节点的next指向指定位置的下一个节点
        current.next.prev = current.prev; // 指定位置下一个节点的prev指向指定位置的前一个节点
    }

    // 查找链表中第一个值为data的节点
    public Node search(int data) {
        Node current = head;
        while (current != null) {
            if (current.data == data) {
                return current; // 找到节点，返回
            }
            current = current.next; // 继续遍历
        }
        return null; // 未找到，返回null
    }

    // 打印链表
    public void printList() {
        Node current = head;
        while (current != null) {
            System.out.print(current.data + " "); // 打印节点数据
            current = current.next; // 继续遍历
        }
        System.out.println(); // 换行
    }
}

双向链表的优点：

  • 能够从头节点和尾节点进行双向遍历。

  • 可以更方便地删除节点，因为可以访问该节点的前一个节点。

双向链表的缺点：

  • 每个节点需要额外的 prev 指针域，导致空间开销更大。

8. 循环链表

循环链表是一种特殊的链表，它的尾节点的指针域指向头节点，形成一个循环。

public class CircularLinkedList {
    // 头节点
    private Node head;

    public CircularLinkedList() {
        head = null;
    }

    // 检查链表是否为空
    public boolean isEmpty() {
        return head == null;
    }

    // 获取链表长度
    public int size() {
        if (isEmpty()) {
            return 0;
        }

        int count = 1;
        Node current = head.next; // 从头节点的下一个节点开始遍历
        while (current != head) { // 当遍历到头节点时停止
            count++;
            current = current.next;
        }
        return count;
    }

    // 在链表头部插入节点
    public void insertAtHead(int data) {
        Node newNode = new Node(data);
        if (isEmpty()) {
            head = newNode;
            newNode.next = head; // 形成循环
        } else {
            newNode.next = head; // 新节点的next指向原头节点
            Node current = head;
            while (current.next != head) { // 遍历到最后一个节点
                current = current.next;
            }
            current.next = newNode; // 最后一个节点的next指向新节点
            head = newNode; // 更新头节点
        }
    }

    // 在链表尾部插入节点
    public void insertAtTail(int data) {
        Node newNode = new Node(data);
        if (isEmpty()) {
            head = newNode;
            newNode.next = head; // 形成循环
        } else {
            Node current = head;
            while (current.next != head) { // 遍历到最后一个节点
                current = current.next;
            }
            current.next = newNode; // 最后一个节点的next指向新节点
            newNode.next = head; // 新节点的next指向头节点
        }
    }

    // 在指定位置插入节点
    public void insertAtIndex(int index, int data) {
        if (index < 0 || index > size()) {
            return; // 非法索引
        }

        if (index == 0) {
            insertAtHead(data); // 如果索引为0，直接调用插入头部方法
            return;
        }

        if (index == size()) {
            insertAtTail(data); // 如果索引等于链表长度，直接调用插入尾部方法
            return;
        }

        Node newNode = new Node(data);
        Node current = head;
        for (int i = 0; i < index - 1; i++) {
            current = current.next; // 遍历到指定位置的前一个节点
        }
        newNode.next = current.next; // 新节点的next指向原指定位置的节点
        current.next = newNode; // 指定位置的前一个节点的next指向新节点
    }

    // 删除链表头部的节点
    public void deleteAtHead() {
        if (isEmpty()) {
            return; // 空链表，无需删除
        }

        if (head.next == head) { // 只有一个节点的情况
            head = null;
            return;
        }

        Node current = head;
        while (current.next != head) { // 遍历到最后一个节点
            current = current.next;
        }
        head = head.next; // 将头节点指向下一个节点
        current.next = head; // 最后一个节点的next指向新的头节点
    }

    // 删除链表尾部的节点
    public void deleteAtTail() {
        if (isEmpty()) {
            return; // 空链表，无需删除
        }

        if (head.next == head) { // 只有一个节点的情况
            head = null;
            return;
        }

        Node current = head;
        while (current.next.next != head) { // 遍历到倒数第二个节点
            current = current.next;
        }
        current.next = current.next.next; // 将倒数第二个节点的next指向倒数第三个节点
    }

    // 删除指定位置的节点
    public void deleteAtIndex(int index) {
        if (index < 0 || index >= size()) {
            return; // 非法索引
        }

        if (index == 0) {
            deleteAtHead(); // 如果索引为0，直接调用删除头部方法
            return;
        }

        if (index == size() - 1) {
            deleteAtTail(); // 如果索引为最后一个节点，直接调用删除尾部方法
            return;
        }

        Node current = head;
        for (int i = 0; i < index - 1; i++) {
            current = current.next; // 遍历到指定位置的前一个节点
        }
        current.next = current.next.next; // 将指定位置的前一个节点的next指向指定位置的下一个节点
    }

    // 查找链表中第一个值为data的节点
    public Node search(int data) {
        if (isEmpty()) {
            return null;
        }

        Node current = head;
        do {
            if (current.data == data) {
                return current; // 找到节点，返回
            }
            current = current.next;
        } while (current != head);

        return null; // 未找到，返回null
    }

    // 打印链表
    public void printList() {
        if (isEmpty()) {
            return;
        }

        Node current = head;
        System.out.print(current.data + " "); // 打印头节点数据
        current = current.next;
        while (current != head) { // 当遍历到头节点时停止
            System.out.print(current.data + " "); // 打印节点数据
            current = current.next;
        }
        System.out.println(); // 换行
    }
}

循环链表的优点：

  • 可以实现一些特殊的数据结构，例如循环队列。

  • 可以更方便地遍历链表，因为可以从任何节点开始遍历。

循环链表的缺点：

  • 维护复杂度更高，需要特别注意循环条件。

9. 总结

链表是一种灵活、动态的数据结构，适合存储动态变化的数据。单向链表、双向链表和循环链表各有优劣，开发者需要根据实际情况选择最合适的链表类型。

需要注意的是，以上代码示例只是简单演示了链表的基本操作，且只使用Java实现，实际应用中可能需要根据具体需求添加其他方法，并进行更完善的错误处理和性能优化。

希望本文能让各位看官更好的掌握链表，感谢各位的观看，下期见，谢谢~

标签：Node,current,head,next,链表,详解,数据结构,节点
From： https://blog.csdn.net/weixin_64178283/article/details/143023729

相关文章

  • 数据结构-B树和B+树
    一、B树一个节点包含多个key-value值假设一棵B树由M个参数构建，我们将其称为M阶B树        每个节点最多有M-1个key-value值，并且key值升序排列，每个节点最多能有M个叉1.1分类二节点三节点四节点五节点key: 给每一个文件进行标号（主键）value: 页（存储数据......
  • 【数据结构】之数组详解
    数组是数据结构中最基础的概念之一，它在编程中被广泛应用。理解数组的工作原理、操作方式以及底层实现，对于我们构建更复杂的数据结构和算法至关重要。本文将从多个角度深入剖析数组，并以Java语言示例进行讲解，帮助你建立对数组的深刻理解。一、什么是数组？数组是一种线性数据结构......
  • Topk问题与堆排序(Java数据结构)
    前言：    接触完堆之后，也逐渐对堆了如指掌，最后再来讨论一下两个问题。    有如下场景：    1、全国有几千所大学，我如何能够快速找出排名前10的大学？    2、我如何对这10所大学排好序？    为了用堆解决问题，接下来我们就来一起学习Top......
  • Java数据结构二叉树面试题精华(画图详解)
    前言：    针对二叉树，因为涉及到递归，需要跟多的练习强化递归的思想，其中也包括需要画图理解一些想不通的问题来提升自己！    一下面这些题为例，一起来提升自己的逻辑思维能力！(可能其中一些题已经写过，但是希望能再写一遍有助于提高代码能力)相同的树：      ......
  • Witness组件详解
    Witness组件详解 ......
  • Nuxt.js 应用中的 app：resolve 事件钩子详解
    title:Nuxt.js应用中的app：resolve事件钩子详解date:2024/10/17updated:2024/10/17author:cmdragonexcerpt:app:resolve是Nuxt.js中的生命周期钩子，在解析app实例后调用。这个钩子允许开发者在应用完全初始化后执行一些自定义操作，比如注册插件、设置中间件或进......
  • 【Docker系列】docker-compose down 命令详解
    ......
  • 翻转链表常用写法
    翻转链表常用写法循环写法classSolution{public:ListNode*reverseList(ListNode*head){ListNode*prev=nullptr,*next=nullptr,*now=head;while(now){next=now->next;now->next=prev;prev=......
  • HttpUtils 详解
    一、详解1.1介绍现如今的Web项目，由服务端向外发起网络请求的场景，基本上随处可见！传统情况下，在服务端代码里访问http服务时，一般会使用JDK的HttpURLConnection或者Apache的HttpClient，不过这种方法使用起来太过繁琐，而且api使用起来非常的复杂，还得操心资源回收。1.2......
  • 解析华为鸿蒙next：Web组件自适应布局与渲染模式详解
    本文旨在深入探讨华为鸿蒙HarmonyOSNext系统（截止目前API12）的技术细节，基于实际开发实践进行总结。主要作为技术分享与交流载体，难免错漏，欢迎各位同仁提出宝贵意见和问题，以便共同进步。本文为原创内容，任何形式的转载必须注明出处及原作者。Web组件大小自适应页面内容布局使用......

赞助商

阅读排行

网站主页关于我们联系我们网站地图

本网站内容转载自其他媒体，侵权联系[admin##ips99.com]。

Copyright © 2020-2023 IPS99 版权所有 IPS99