代码随想录训练营｜Day 23｜669，108，538，总结

标签：right 669 23 tree 随想录 low null root left

669. Trim a Binary Search Tree

Given the root of a binary search tree and the lowest and highest boundaries as low and high, trim the tree so that all its elements lies in [low, high]. Trimming the tree should not change the relative structure of the elements that will remain in the tree (i.e., any node's descendant should remain a descendant). It can be proven that there is a unique answer.

Return the root of the trimmed binary search tree. Note that the root may change depending on the given bounds.

Example 1:

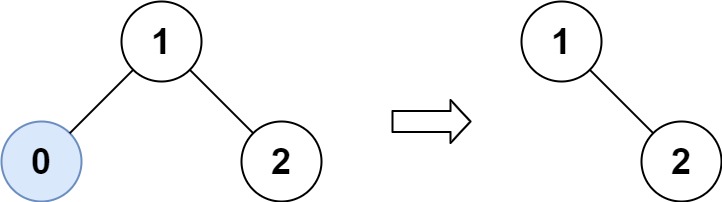
Input: root = [1,0,2], low = 1, high = 2
Output: [1,null,2]

Example 2:

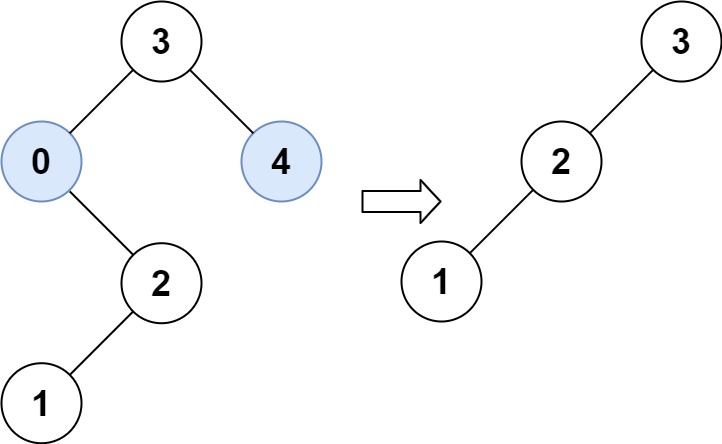
Input: root = [3,0,4,null,2,null,null,1], low = 1, high = 3
Output: [3,2,null,1]

Constraints:

• The number of nodes in the tree is in the range [1, 104].

• 0 <= Node.val <= 104

• The value of each node in the tree is unique.

• root is guaranteed to be a valid binary search tree.

• 0 <= low <= high <= 104

• 如果root（当前节点）的元素小于low的数值，那么应该递归右子树，并返回右子树符合条件的头结点。

• 如果root(当前节点)的元素大于high的，那么应该递归左子树，并返回左子树符合条件的头结点。

• 将下一层处理完左子树的结果赋给root->left，处理完右子树的结果赋给root->right。

class Solution {
    public TreeNode trimBST(TreeNode root, int low, int high) {
        if (root == null) {
            return null;
        }
        if (root.val < low) {
            return trimBST(root.right, low, high);
        }
        if (root.val > high) {
            return trimBST(root.left, low, high);
        }
        // root在[low,high]范围内
        root.left = trimBST(root.left, low, high);
        root.right = trimBST(root.right, low, high);
        return root;
    }
}

For Future References

题目链接：https://leetcode.com/problems/trim-a-binary-search-tree/

文章讲解：https://programmercarl.com/0669.修剪二叉搜索树.html

---

108. Convert Sorted Array to Binary Search Tree

Given an integer array nums where the elements are sorted in ascending order, convert it to a height-balanced binary search tree.

A height-balanced binary tree is a binary tree in which the depth of the two subtrees of every node never differs by more than one.

Example 1:

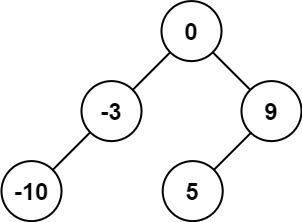
Input: nums = [-10,-3,0,5,9]
Output: [0,-3,9,-10,null,5]
Explanation: [0,-10,5,null,-3,null,9] is also accepted:

Example 2:

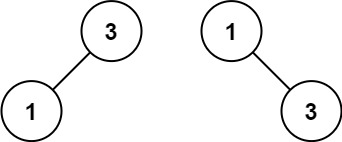
Input: nums = [1,3]
Output: [3,1]
Explanation: [1,null,3] and [3,1] are both height-balanced BSTs.

Constraints:

• 1 <= nums.length <= 104
• 104 <= nums[i] <= 104
• nums is sorted in a strictly increasing order.

用递归函数的返回值来构造中节点的左右孩子。
首先取数组中间元素的位置
以中间位置的元素构造节点
接着划分区间，root的左孩子接住下一层左区间的构造节点，右孩子接住下一层右区间构造的节点。
int mid = left + ((right - left) / 2);的写法相当于是如果数组长度为偶数，中间位置有两个元素，取靠左边的。

//递归: 左闭右闭 [left,right]
class Solution {
	public TreeNode sortedArrayToBST(int[] nums) {
		TreeNode root = traversal(nums, 0, nums.length - 1);
		return root;
	}

	// 左闭右闭区间[left, right)
	private TreeNode traversal(int[] nums, int left, int right) {
		if (left > right) return null;

		int mid = left + ((right - left) >> 1);
		TreeNode root = new TreeNode(nums[mid]);
		root.left = traversal(nums, left, mid - 1);
		root.right = traversal(nums, mid + 1, right);
		return root;
	}
}

For Future References

题目链接：https://leetcode.com/problems/convert-sorted-array-to-binary-search-tree/

文章讲解：https://programmercarl.com/0108.将有序数组转换为二叉搜索树.html

---

538. Convert BST to Greater Tree

Given the root of a Binary Search Tree (BST), convert it to a Greater Tree such that every key of the original BST is changed to the original key plus the sum of all keys greater than the original key in BST.

As a reminder, a binary search tree is a tree that satisfies these constraints:

• The left subtree of a node contains only nodes with keys less than the node's key.
• The right subtree of a node contains only nodes with keys greater than the node's key.
• Both the left and right subtrees must also be binary search trees.

Example 1:

Input: root = [4,1,6,0,2,5,7,null,null,null,3,null,null,null,8]
Output: [30,36,21,36,35,26,15,null,null,null,33,null,null,null,8]

Example 2:

Input: root = [0,null,1]
Output: [1,null,1]

Constraints:

• The number of nodes in the tree is in the range [0, 104].
• 104 <= Node.val <= 104
• All the values in the tree are unique.
• root is guaranteed to be a valid binary search tree.

从树中可以看出累加的顺序是右中左，所以我们需要反中序遍历这个二叉树，然后顺序累加就可以了
需要一个pre指针记录当前遍历节点cur的前一个节点，这样才方便做累加
要右中左来遍历二叉树， 中节点的处理逻辑就是让cur的数值加上前一个节点的数值。

class Solution {
    int sum;
    public TreeNode convertBST(TreeNode root) {
        sum = 0;
        convertBST1(root);
        return root;
    }

    // 按右中左顺序遍历，累加即可
    public void convertBST1(TreeNode root) {
        if (root == null) {
            return;
        }
        convertBST1(root.right);
        sum += root.val;
        root.val = sum;
        convertBST1(root.left);
    }
}

For Future References

题目链接：https://leetcode.com/problems/convert-bst-to-greater-tree/

文章讲解：https://programmercarl.com/0538.把二叉搜索树转换为累加树.html

---

Summary of Binary Trees

涉及到二叉树的构造，无论普通二叉树还是二叉搜索树一定前序，都是先构造中节点。

求普通二叉树的属性，一般是后序，一般要通过递归函数的返回值做计算。

求二叉搜索树的属性，一定是中序了，要不白瞎了有序性了。

For Future References

文章讲解：https://programmercarl.com/二叉树总结篇.html#二叉树的理论基础

标签：right,669,23,tree,随想录,low,null,root,left
From： https://www.cnblogs.com/bluesociety/p/16786679.html