51.N-Queens

The n-queens puzzle is the problem of placing n queens on an n x n chessboard such that no two queens attack each other.

Given an integer n, return all distinct solutions to the n-queens puzzle. You may return the answer in any order.

Each solution contains a distinct board configuration of the n-queens' placement, where 'Q' and '.' both indicate a queen and an empty space, respectively.

Example 1:

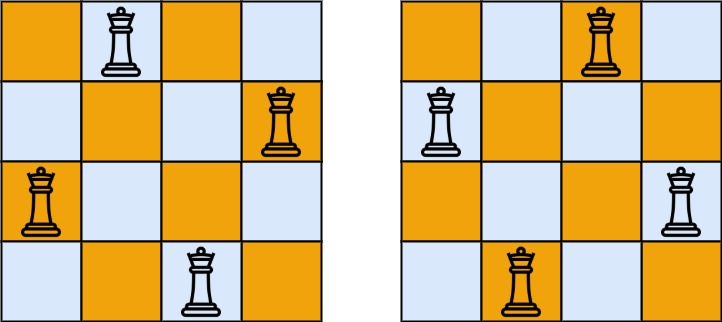
Input: n = 4
Output: [[".Q..","...Q","Q...","..Q."],["..Q.","Q...","...Q",".Q.."]]
Explanation: There exist two distinct solutions to the 4-queens puzzle as shown above

Example 2:

Input: n = 1
Output: [["Q"]]

public static List<List<String>> solveNQueens(int n) {
    List<List<String>> res = new ArrayList<>();
    int[] queenList = new int[n]; //第i个位置存放的数表示row行时，Q的列，如果queenList[0] = 2，表示第0行的第二列是Q
    placeQueen(queenList, 0, n, res);//在第0行放Q
    return res;
}

private static void placeQueen(int[] queenList, int row, int n, List<List<String>> res) {
    //如果已经填满，就生成结果
    if (row == n) {
        ArrayList<String> list = new ArrayList<>();
        for (int i = 0; i < n; i++) {
            String str = "";
            for (int col = 0; col < n; col++){
                if(queenList[i] == col) {
                    str += "Q";
                } else {
                    str += ".";
                }
            }
            list.add(str);
        }
        res.add(list);
    }
    for (int col = 0; col < n; col++) {//循环每一列
        if (isValid(queenList, row, col)) { //如果在该列放入Q不冲突的话
            queenList[row] = col;
            placeQueen(queenList, row + 1, n, res);
        }
    }
}

private static boolean isValid(int[] queenList, int row, int col) {
    for (int i = 0; i < row; i++) {
        int pos = queenList[i];
        if (pos == col) { //和新加入的Q处于同一列
            return false;
        }
        if (pos + row - i == col) { //在新加入的Q的右对角线上
            return false;
        }
        if (pos - row + i == col) { //在新加入的Q的左对角线上
            return false;
        }
    }
    return true;
}